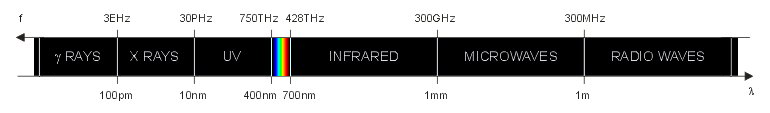
The electromagnetic spectrum (not to scale).
The visible spectrum is a very small portion of the electromagnetic spectrum that can be seen by the human eye. This is summarized in the figure below, which is not to scale. The exact boundaries of each type of wave are not precisely defined and often different authors have slightly different values. This is not really relevant since this classification is just indicative.

The electromagnetic spectrum (not to scale).
As frequency increases the wavelength becomes smaller and the energy associated to each photon increases. Visible light, infrared and radiation with longer wavelengths are non-ionizing radiations. UV light, X rays, gamma rays and radiations with shorter wavelength are ionizing radiations, meaning that each photon carries enough energy to ionize matter. When a ionizing photon with enough energy impinges on an atom or on a molecule, it can knock off one electron from it. Having lost an electron, the atom (or molecule) becomes electrically charged and is called an ion. Because of their electrical charge, ions are chemically very active and tend to react with nearby molecules. The limit between ionizing and non-ionizing radiations is located somewhere in the UV region, but it's not clearly defined and somehow fuzzy. A photon energy1 of 10 eV (corresponding to a wavelength of 124 nm) is often considered the threshold between ionizing and non-ionizing radiation, but there is no consensus on this topic.
The wavelengths2 of the visible spectrum are usually between 400 nm and 700 nm. The energy carried by each visible photon is between 3.1 eV and 1.8 eV respectively and the frequency is in the 750 THz to 428 THz range
The sensitivity of the eye to wavelengths beyond this range drops dramatically and they are represented as black in the figure below. The maximum sensitivity is usually between 500 and 550 nm.
The perceived color dependens on the wavelength and the table below will give a rough idea. Of course, this varies from one person to another.
| Red | 700...630 nm |
| Orange | 630...600 nm |
| Yellow | 600...570 nm |
| Green | 570...530 nm |
| Cyan | 530...490 nm |
| Indigo | 490...450 nm |
| Blue | 450...400 nm |
You may be surprised that the color called violet actually looks blue and that there is no purple nor pink: this is because in the 17th century when Newton discovered the spectrum of the sunlight, the names of the colors corresponded to slightly different hues. He called violet what we would call blue today and blue what we would call cyan. You may also think at the famous poem "roses are red and violets are blue", the hue of the violets is actually blue...
Purple and pink, on the other hand, are colors that do not correspond to an unique wavelength, they are perceived by eye when both blue and red light are superposed. Since blue and red are at opposite sides of the visible spectrum, no single wavelength will appear purple or pink.
Talking about missing colors, there is no brown nor grey light neither: these colors are just perceived when compared with other brighter colors, gray is a dim white and brown is a dim yellow/orange.
Wavelengths shorter than 390 nm are part of the UV spectrum and are not visible; UV meaning ultra violet, beyond violet (meaning blue). Wavelengths longer than 750 nm are part of the IR spectrum and are not visible neither; IR meaning infrared, below red. It's not possible to give a precise boundary between visible and invisible wavelengths since that's very subjective: some people may still see some light at 720 nm and some others may not.
White light, a superposition of all colors, can be decomposed with a prism to observe its spectrum. Just shining light at a prism is not enough; the light has to go through a narrow slit to let only a small ray pass through. The quality of this slit is very important for the precision of the result. In the example below, the slit is just a crude cut in a piece of cardboard and makes a very rough spectrometer, but still enough to observe a "rainbow".

Visible spectrum of white light observed with a prism.
| [1] | Warren J. Smith. Modern Optical Engineering - The Design of Optical Systems. 3rd Edition, McGraw-Hill, 2000, section 1.1. |
| [2] | Eugene Hecht. Optics. 4th Edition, Addison Wesley, 2002, section 3.6. |
| 1: | The energy of a photon of a given wavelength id given by Planck's law E = hν or E = hc/λ where E is the photon energy in electronvolt (eV), h is Plank's constant 4.135'667'517·10−15 eV·s, c is the speed of light 299'792'458 m/s, ν is the photon frequency in Hertz and λ is the photon wavelength in meters. |
| 2: |
1 nm = 10–9 m = 0.000'000'001 m 1 THz = 1012 Hz = 1'000'000'000'000 Hz |
| Home | Optics | Page hits: 260358 | Created: 01.2004 | Last update: 01.2018 |